General Information
Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
Test Item Specifications
The center of dilation must be given.
Neutral
Students will use similarity to derive the fact that the length of the arc
intercepted by an angle is proportional to the radius, and define the
radian measure as the constant of proportionality.
Students will apply similarity to solve problems that involve the
length of the arc intercepted by an angle and the radius of a circle.
Students will derive the formula for the area of a sector.
Students will use the formula for the area of a sector to solve
problems.
Items may be set in a real-world or mathematical context
Items may require the student to use or choose the correct unit of
measure.
Sample Test Items (2)
| Test Item # | Question | Difficulty | Type |
| Sample Item 1 | Circle Q has a radius,r, in units. The measure of
A. Create an expression using r and x that can be used to find the length of B. Then, create an expression that could be used to find the length of |
N/A | EE: Equation Editor |
| Sample Item 2 | Natasha's classmates have ordered her a cookie pizza for her birthday celebration. To share it among the class, they will be slicing the cookie into equal pieces. They want everyone to have an icing packet to add to his or her slice. In order to approximate how much icing each person will need, they want to know the area of each slice of cookie. The following table lists the radius of the cookie pizza and the area of some of the possible divisions of the cookie.
This question has three parts. Part A. What is the relationship between the area of a whole cookie with a radius of 6 units and the area of a portion whose central angle measures k degrees? Part B. Using the information in the table, click on the blank to complete the equation for finding the area of a portion of a cookie with a radius of 6 units when given the measure of a central angle, in degrees, of the portion. Part C. Click on the blank to complete the formula that can be used to find the area of any portion of a circle with radius w and a central angle of z degrees.
|
N/A | : Multiple Types |
Related Courses
| Course Number1111 | Course Title222 |
| 1200400: | Foundational Skills in Mathematics 9-12 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1206310: | Geometry (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1206320: | Geometry Honors (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1206315: | Geometry for Credit Recovery (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 7912065: | Access Geometry (Specifically in versions: 2015 - 2022, 2022 and beyond (current)) |
Related Resources
Formative Assessments
| Name | Description |
| Deriving the Sector Area Formula | Students are asked to write a formula to find the area of a sector of a circle and then explain and justify that formula. |
| Arc Length and Radians | Students are asked to explain why the length of an arc intercepted by an angle is proportional to the radius and then explain how that proportionality leads to a definition of the radian measure of an angle. |
| Sector Area | Students are asked to find the areas of sectors in two different circles. |
| Arc Length | Students are asked to find the lengths of arcs in two different circles. |
Lesson Plans
| Name | Description |
| My Favorite Slice | The lesson introduces students to sectors of circles and illustrates ways to calculate their areas. The lesson uses pizzas to incorporate a real-world application for the of area of a sector. Students should already know the parts of a circle, how to find the circumference and area of a circle, how to find an arc length, and be familiar with ratios and percentages. |
| Sectors of Circles | This lesson unit is intended to help you assess how well students are able to solve problems involving area and arc length of a sector of a circle using radians. It assumes familiarity with radians and should not be treated as an introduction to the topic. This lesson is intended to help you identify and assist students who have difficulties in computing perimeters, areas, and arc lengths of sectors using formulas and finding the relationships between arc lengths, and areas of sectors after scaling. |
| The Grass is Always Greener | The lesson introduces area of sectors of circles then uses the areas of circles and sectors to approximate area of 2-D figures. The lesson culminates in using the area of circles and sectors of circles as spray patterns in the design of a sprinkler system between a house and the perimeter of the yard (2-D figure). |
Problem-Solving Tasks
| Name | Description |
| Two Wheels and a Belt | This task combines two skills: making use of the relationship between a tangent segment to a circle and the radius touching that tangent segment, and computing lengths of circular arcs given the radii and central angles. |
| Setting Up Sprinklers | This modeling task involves several different types of geometric knowledge and problem-solving: finding areas of sectors of circles, using trigonometric ratios to solve right triangles, and decomposing a complicated figure involving multiple circular arcs into parts whose areas can be found. |
Text Resource
| Name | Description |
| Why Tau Trumps Pi | This informational text resource is intended to support reading in the content area. The author tries to convince the reader that two pi, or tau, occurs more often in mathematics than pi by itself. The author provides several examples and indicates the history behind society's choice of pi rather than tau. |
Tutorial
| Name | Description |
| Pi Fight: Pi vs. Tau | Click "View Site" to open a full-screen version. This tutorial is designed to help secondary math teachers learn how to integrate literacy skills within their curriculum. This tutorial focuses on evaluating the reasoning and evidence of an argumentative claim. The focus on literacy across content areas is designed to help students independently build knowledge in different disciplines through reading and writing. |
Student Resources
Problem-Solving Tasks
| Name | Description |
| Two Wheels and a Belt: | This task combines two skills: making use of the relationship between a tangent segment to a circle and the radius touching that tangent segment, and computing lengths of circular arcs given the radii and central angles. |
| Setting Up Sprinklers: | This modeling task involves several different types of geometric knowledge and problem-solving: finding areas of sectors of circles, using trigonometric ratios to solve right triangles, and decomposing a complicated figure involving multiple circular arcs into parts whose areas can be found. |
Parent Resources
Problem-Solving Tasks
| Name | Description |
| Two Wheels and a Belt: | This task combines two skills: making use of the relationship between a tangent segment to a circle and the radius touching that tangent segment, and computing lengths of circular arcs given the radii and central angles. |
| Setting Up Sprinklers: | This modeling task involves several different types of geometric knowledge and problem-solving: finding areas of sectors of circles, using trigonometric ratios to solve right triangles, and decomposing a complicated figure involving multiple circular arcs into parts whose areas can be found. |

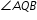 is xº, as shown.
is xº, as shown.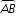 , in units.
, in units.