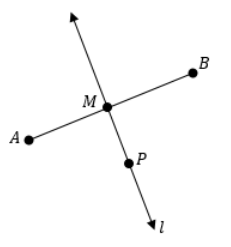
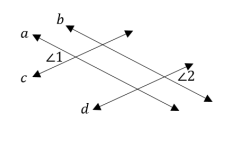
Prove relationships and theorems about lines and angles. Solve mathematical and real-world problems involving postulates, relationships and theorems of lines and angles.
: Postulates, relationships and theorems include vertical angles are congruent; when a transversal crosses parallel lines, the consecutive angles are supplementary and alternate (interior and exterior) angles and corresponding angles are congruent; points on a perpendicular bisector of a line segment are exactly those equidistant from the segment’s endpoints.
| Name |
Description |
| Finding Angle Measures - 1 | Students are asked to find the measures of angles formed by three concurrent lines and to justify their answers. |
| Finding Angle Measures - 3 | Students are asked to find the measures of angles formed by two parallel lines and two transversals. |
| Finding Angle Measures - 2 | Students are asked to find the measures of angles formed by two parallel lines and a transversal. |
| Camping Calculations | Students are asked to find the measure of an angle formed by the support poles of a tent using the properties of geometric shapes. |
| Same Side Interior Angles | Students are asked to describe and justify the relationship between same side interior angles. |
| Justifying Angle Relationships | Students are asked to describe and justify the relationship between corresponding angles and alternate interior angles. |
| Proving the Alternate Interior Angles Theorem | In a diagram involving two parallel lines and a transversal, students are asked to use rigid motion to prove that alternate interior angles are congruent. |
| Constructions for Parallel Lines | Students are asked to construct a line parallel to a given line through a given point. |
| Equidistant Points | Students are asked to prove that a point on the perpendicular bisector of a line segment is equidistant from the endpoints of the segment. |
| Proving the Vertical Angles Theorem | Students are asked to identify a pair of vertical angles in a diagram and then prove that they are congruent. |
| Name |
Description |
| Triangle Mid-Segment Theorem | The Triangle Mid-Segment Theorem is used to show the writing of a coordinate proof clearly and concisely. |
| Proof of Quadrilaterals in Coordinate Plane | This lesson is designed to instruct students on how to identify special quadrilaterals in the coordinate plane using their knowledge of distance formula and the definitions and properties of parallelograms, rectangles, rhombuses, and squares. Task cards, with and without solution-encoded QR codes, are provided for cooperative group practice. The students will need to download a free "QR Code Reader" app onto their SmartPhones if you choose to use the cards with QR codes. |
| To Be or Not to Be a Parallelogram | Students apply parallelogram properties and theorems to solve real world problems. The acronym, P.I.E.S. is introduced to support a problem solving strategy, which involves drawing a Picture, highlighting important Information, Estimating and/or writing equation, and Solving problem. |
| Parallel Thinking Debate | Students prove theorems related to parallel lines using vertical, corresponding, and alternate interior angles. |
| Vertical Angles: Proof and Problem-Solving | Students will explore the relationship between vertical angles and prove the Vertical Angle Theorem. They will use vertical angle relationships to calculate other angle measurements. |
| Diagonally Half of Me! | This lesson is an exploration activity assisting students prove that diagonals of parallelograms bisect each other. It allows them to compare other quadrilaterals with parallelograms in order to make conjectures about the diagonals of parallelograms. |
| Who Am I?: Quadrilaterals | Students will use formulas they know (distance, midpoint, and slope) to classify quadrilaterals. |
| Proving and Using Congruence with Corresponding Angles | Students, will prove that corresponding angles are congruent. Directions for using GeoGebra software to discover this relationship is provided. |
| Proving Parallelograms Algebraically | This lesson reviews the definition of a parallelogram and related theorems. Students use these conditions to algebraically prove or disprove a given quadrilateral is a parallelogram. |
| How Much Proof Do We Need? | Students determine the minimum amount of information needed to prove that two triangles are similar. |
| Proving quadrilaterals algebrically using slope and distance formula | Working in groups, students will prove the shape of various quadrilaterals using slope, distance formula, and polygon properties. They will then justify their proofs to their classmates. |
| Quadrilaterals and Coordinates | In this lesson, students will use coordinates to algebraically prove that quadrilaterals are rectangles, parallelograms, and trapezoids. A through introduction to writing coordinate proofs is provided as well as plenty of practice. |
| Observing the Centroid | Students will construct the medians of a triangle then investigate the intersections of the medians. |
| Determination of the Optimal Point | Students will use dynamic geometry software to determine the optimal location for a facility under a variety of scenarios. The experiments will suggest a relation between the optimal point and a common concept in geometry; in some cases, there will be a connection to a statistical concept. Algebra can be used to verify some of the conjectures. |
| Proving Quadrilaterals | This lesson provides a series of assignments for students at the Getting Started, Moving Forward, and Almost There levels of understanding for the Mathematics Formative Assessment System (MFAS) Task Describe the Quadrilateral (CPALMS Resource ID#59180). The assignments are designed to "move" students from a lower level of understanding toward a complete understanding of writing a coordinate proof involving quadrilaterals. |
| The Centroid | Students will construct the centroid of a triangle using graph paper or GeoGebra in order to develop conjectures. Then students will prove that the medians of a triangle actually intersect using the areas of triangles. |
| What's the Point? | Students will algebraically find the missing coordinates to create a specified quadrilateral using theorems that represent them, and then algebraically prove their coordinates are correct.
Note: This is not an introductory lesson for this standard. |
| Polygon...Prove it | While this is an introductory lesson on the standard, students will enjoy it, as they play "Speed Geo-Dating" during the Independent practice portion. Students will use algebra and coordinates to prove rectangles, rhombus, and squares. Properties of diagonals are not used in this lesson. |
| Parallel Lines | Students will prove that alternate interior angles and corresponding angles are congruent given two parallel lines and a traversal. Students will use GeoGebra to explore real-world images to prove their line segments are parallel. |
| Let's Prove the Pythagorean Theorem | Students will use Triangle Similarity to derive the proof of the Pythagorean Theorem and apply this method to develop the idea of the geometric mean with respect to the relationships in right triangles. |
| Evaluating Statements About Length and Area | This lesson unit is intended to help you assess how well students can understand the concepts of length and area, use the concept of area in proving why two areas are or are not equal and construct their own examples and counterexamples to help justify or refute conjectures. |
| Scientific calculations from a distant planet | Students will act as mathematicians and scientists as they use models, observations and space science concepts to perform calculations and draw inferences regarding a fictional solar system with three planets in circular orbits around a sun. Among the calculations are estimates of the size of the home planet (using a method more than 2000 years old) and the relative distances of the planets from their sun. |
| Special Angle Pairs Discovery Activity | This lesson uses a discovery approach to identify the special angles formed when a set of parallel lines is cut by a transversal. During this lesson, students identify the angle pair and the relationship between the angles. Students use this relationship and special angle pairs to make conjectures about which angle pairs are considered special angles. |
| Help me Find my Relationship! | In this lesson, students will investigate the relationship between angles when parallel lines are cut by a transversal. Students will identify angles, and find angle measures, and they will use the free application GeoGebra (see download link under Suggested Technology) to provide students with a visual representation of angle relationships. |
| An Investigation of Angle Relationships Formed by Parallel Lines and a Transversal Using GeoGebra | In this lesson, students will discover angle relationships formed when two parallel lines are cut by a transversal (corresponding, alternate interior, alternate exterior, same-side interior, same-side exterior). They will establish definitions and identify whether these angle pairs are supplementary or congruent. |
| Accurately Acquired Angles | Students will start the lesson by playing a game to review angle pairs formed by two lines cut by a transversal. Once students are comfortable with the angle pairs the teacher will review the relationships that are created once the pair of lines become parallel. The teacher will give an example of a proof using the angle pairs formed by two parallel lines cut by a transversal. The students are then challenged to prove their own theorem in groups of four. The class will then participate in a Stay and Stray to view the other group's proofs. The lesson is wrapped up through white board questions answered within groups and then as a whole class. |
| What's the Point? Part 1 | This is a patty paper-folding activity where students measure and discover the properties of the point of concurrency of the perpendicular bisectors of the sides of a triangle. |
| What's Your Angle? | Through a hands-on-activity and guided practice, students will explore parallel lines intersected by a transversal and the measurements and relationships of the angles created. They will solve for missing measurements when given a single angle's measurement. They will also use the relationships between angles to set up equations and solve for a variable. |
| Name |
Description |
| Copying an Angle and Parallel Lines | Students will learn how to copy a given angle and use that skill to construct parallel lines. They will explore triangle congruence proofs and identify special angle pairs formed by parallel lines and transversals. |
| Introducing Construction Tools and Basic Geometric Constructions | Students will learn to use basic geometric construction tools to copy a segment and an angle, then apply these skills repeatedly to construct a rhombus. The definition of a parallelogram is verified. |
| Euler Line | Students will construct bisectors of segments to verify definitions and constructions of midpoints, medians, altitudes, the orthocenter, centroid, circumcenter, and the converse of the perpendicular bisector theorem, leading to the discovery of the Euler Line. |
| Related Constructions to the Perpendicular Bisector | Students will use the construction of a perpendicular bisector to verify the Triangle Inequality Theorem. They will relate the radius of the compass needed to create intersecting arcs to the side-length conditions required for a triangle to exist. |
| Construction of the Bisector of a Segment | Students will construct the perpendicular bisector of a segment to verify the construction of a rhombus as a parallelogram. Similarities and differences between a segment bisector and perpendicular bisector are revealed. |
| Parallel Lines and Partitions | Students will construct congruent segments on one ray of an angle and learn how to use a geometry set to create parallel lines to partition the other ray into a given ratio. The results are verified using corresponding angles and similar triangles. |
| The Golden Ratio | Students will use segment constructions to verify and demonstrate the golden ratio. These proportional relationships connect the golden ratio to the ancient Greek ideal of beauty, balance, and harmony. |
| Name |
Description |
| Tile Patterns I: octagons and squares | Students use interior and exterior angles to to verify attributes of an octagon and square. Students are given a tile pattern involving congruent regular octagons and squares. |
| Points equidistant from two points in the plane | This task asks students to show how certain points on a plane are equidistant to points on a segment when placed on a perpendicular bisector. |
| Reflected Triangles | This task asks students to use a straightedge and compass to construct the line across which a triangle is reflected. |
| Tangent Lines and the Radius of a Circle | This problem solving task challenges students to find the perpendicular meeting point of a segment from the center of a circle and a tangent. |
| Find the Angle | Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. |
| Find the Missing Angle | This task provides students the opportunity to see how the mathematical ideas embedded in the standards and clusters mature over time. The task uses facts about supplementary, complementary, vertical, adjacent, and alternate interior angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure. It is a good introduction to writing paragraphs, 2-column, and/or flow chart proofs. |
| Is This a Rectangle? | The goal of this task is to provide an opportunity for students to apply a wide range of ideas from geometry and algebra in order to show that a given quadrilateral is a rectangle. Creativity will be essential here as the only given information is the Cartesian coordinates of the quadrilateral's vertices. Using this information to show that the four angles are right angles will require some auxiliary constructions. Students will need ample time and, for some of the methods provided below, guidance. The reward of going through this task thoroughly should justify the effort because it provides students an opportunity to see multiple geometric and algebraic constructions unified to achieve a common purpose. The teacher may wish to have students first brainstorm for methods of showing that a quadrilateral is rectangle (before presenting them with the explicit coordinates of the rectangle for this problem): ideally, they can then divide into groups and get to work straightaway once presented with the coordinates of the quadrilateral for this problem. |
| Name |
Description |
| Tile Patterns I: octagons and squares: | Students use interior and exterior angles to to verify attributes of an octagon and square. Students are given a tile pattern involving congruent regular octagons and squares. |
| Points equidistant from two points in the plane: | This task asks students to show how certain points on a plane are equidistant to points on a segment when placed on a perpendicular bisector. |
| Reflected Triangles: | This task asks students to use a straightedge and compass to construct the line across which a triangle is reflected. |
| Tangent Lines and the Radius of a Circle: | This problem solving task challenges students to find the perpendicular meeting point of a segment from the center of a circle and a tangent. |
| Find the Angle: | Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. |
| Find the Missing Angle: | This task provides students the opportunity to see how the mathematical ideas embedded in the standards and clusters mature over time. The task uses facts about supplementary, complementary, vertical, adjacent, and alternate interior angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure. It is a good introduction to writing paragraphs, 2-column, and/or flow chart proofs. |
| Is This a Rectangle?: | The goal of this task is to provide an opportunity for students to apply a wide range of ideas from geometry and algebra in order to show that a given quadrilateral is a rectangle. Creativity will be essential here as the only given information is the Cartesian coordinates of the quadrilateral's vertices. Using this information to show that the four angles are right angles will require some auxiliary constructions. Students will need ample time and, for some of the methods provided below, guidance. The reward of going through this task thoroughly should justify the effort because it provides students an opportunity to see multiple geometric and algebraic constructions unified to achieve a common purpose. The teacher may wish to have students first brainstorm for methods of showing that a quadrilateral is rectangle (before presenting them with the explicit coordinates of the rectangle for this problem): ideally, they can then divide into groups and get to work straightaway once presented with the coordinates of the quadrilateral for this problem. |
| Name |
Description |
| Tile Patterns I: octagons and squares: | Students use interior and exterior angles to to verify attributes of an octagon and square. Students are given a tile pattern involving congruent regular octagons and squares. |
| Points equidistant from two points in the plane: | This task asks students to show how certain points on a plane are equidistant to points on a segment when placed on a perpendicular bisector. |
| Reflected Triangles: | This task asks students to use a straightedge and compass to construct the line across which a triangle is reflected. |
| Tangent Lines and the Radius of a Circle: | This problem solving task challenges students to find the perpendicular meeting point of a segment from the center of a circle and a tangent. |
| Find the Angle: | Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. |
| Find the Missing Angle: | This task provides students the opportunity to see how the mathematical ideas embedded in the standards and clusters mature over time. The task uses facts about supplementary, complementary, vertical, adjacent, and alternate interior angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure. It is a good introduction to writing paragraphs, 2-column, and/or flow chart proofs. |
| Is This a Rectangle?: | The goal of this task is to provide an opportunity for students to apply a wide range of ideas from geometry and algebra in order to show that a given quadrilateral is a rectangle. Creativity will be essential here as the only given information is the Cartesian coordinates of the quadrilateral's vertices. Using this information to show that the four angles are right angles will require some auxiliary constructions. Students will need ample time and, for some of the methods provided below, guidance. The reward of going through this task thoroughly should justify the effort because it provides students an opportunity to see multiple geometric and algebraic constructions unified to achieve a common purpose. The teacher may wish to have students first brainstorm for methods of showing that a quadrilateral is rectangle (before presenting them with the explicit coordinates of the rectangle for this problem): ideally, they can then divide into groups and get to work straightaway once presented with the coordinates of the quadrilateral for this problem. |