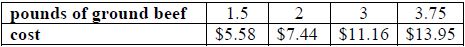Connecting Benchmarks/Horizontal Alignment
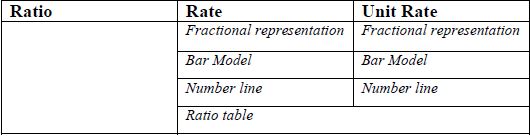
Terms from the K-12 Glossary
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
In grade 5, students represented the division of two whole numbers as a fraction. In doing this, students started to work with a ratio relationship that relates parts to wholes. In grade 6, students extend this concept to include rates, which are ratios between quantities that are most often in different units. Students use ratio relationships to describe unit rates and percentage relationship and use the division of positive rational numbers to calculate unit rates from rates. In grade 7, students learn that a unit rate is the same as a constant of proportionality in a proportional relationship between two variables.
- Instruction connects rate and unit rate to student understanding of equivalent fractions from elementary school in both numeric and picture or model forms. Students can use the models to represent the situations in different ways (MTR.5.1).
- Allow student flexibility in accepting both simplified and non-simplified responses for rates unless unit rate is the specified or expected form.
Common Misconceptions or Errors
- Students may incorrectly identify what is being compared or the order of quantities being compared by the rate.
- Students may have difficulty connecting a unit rate, which is represented by a single number, to a ratio or non-unit rate, which may be represented by two numbers.
Strategies to Support Tiered Instruction
- Instruction includes the use of manipulatives and models to represent the provided rates and then to use multiplicative reasoning to determine the rate of one unit. Manipulatives and models include snap cubes, marbles, bar models, number lines or rate tables to help visually represent the relationship.
- Instruction includes the use of manipulatives to allow for students to explore the meaning of a unit rate. The teacher should provide two different counters to represent a rate equivalent to a whole number unit rate and then co-model the division of the counters into equal groups to determine how many counters of one color are needed to represent a single counter of the other color.
- For example: At the grocery store, you paid $9.00 for 3 pounds of apples. What is the unit price paid per pound of apples?
Instructional Tasks
Instructional Task 1 (MTR.6.1, MTR.7.1)
When buying ground beef for hamburgers, there are several packages from which to choose as shown in the table below.
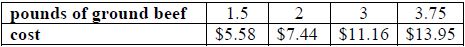
- Part A. Predict how much it would cost for a pound of ground beef. Explain why your prediction is reasonable.
- Part B. What is the unit cost of ground beef? Does the unit cost differ by the package size at this store?
Instructional Task 2 (MTR.4.1)
The Jones family is planning on expanding their garden so that they can plant more vegetables. The ratio of the area of the old garden to the area of the new garden is 4¼:8 ¾. Convert this ratio to a unit rate and explain what it means in this context.
Instructional Task 3 (MTR.2.1, MTR.4.1, MTR.5.1) Stephen is tracking the number of miles traveled to the number of minutes passed while traveling. He discovered that in 8 minutes he traveled 6 miles.
- Part A. In your group, use the chart below to determine the rate and unit rate in miles per minute.
- Part B. Which form would be most efficient for this context? Why?
Instructional Items
Instructional Item 1 At the grocery store, you paid $9.87 for 3.3 pounds of apples. What is the unit price paid per pound of apples? (Round to the nearest cent.)
Instructional Item 2 Brenda wants to buy one of the three cereals listed below. Determine which box is the best buy. Show and explain how you determined this.
- 16 ounces of Frosted Flurries for $3.50
- 12.4 ounces of Chocolate O’s for $2.42
- 11.5 ounces of Cinnamon Grahams for $2.35
*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.
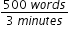 which is equivalent to the unit rate of
which is equivalent to the unit rate of 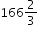 words per minute.
words per minute.
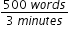 which is equivalent to the unit rate of
which is equivalent to the unit rate of 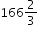 words per minute.
words per minute.