Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
The purpose of this benchmark is for students to plot and order fractions with the same numerator (e.g.,
,
,
) or fractions with the same denominator (e.g.,
,
,
) to compare them
by their location on a number line.
- During instruction, teachers can provide students opportunities to practice using the number line, which will assist students with understanding the difference in size when fractions have the same numerator (the size of the parts) and with comparing fractions with the same denominator (number of parts) (MTR.2.1).
- Through making connections to rulers, students see that appropriately scaled number lines allow for comparisons of fraction size. Students should also utilize open number lines to practice creating their own appropriately scaled number lines (MTR.2.1).
- Through making connections to rulers, students see that appropriately scaled number lines allow for comparisons of fraction size. Students should also utilize open number lines to practice creating their own appropriately scaled number lines (MTR.2.1). Instruction demonstrates that number lines can model fractional values as intervals that can be counted using unit fractions. For example, on a number line is represented by 5 units from 0
that are each one-third in length. Second, number lines help students see comparisons of fractions to the same whole and will continue as students compare fractions with different numerators and denominators in Grade 4. Finally, number lines reinforce Clarification 3 for MA.3.FR.1.3, that fractions are numbers (MTR.2.1, MTR.5.1).
Common Misconceptions or Errors
- Students can be confused that when numerators are the same in fractions, larger denominators represent smaller pieces, and smaller denominators represent larger pieces.
- When fraction comparisons are made using area models, students may be confused that the size of the whole for each model must be the same size.
Strategies to Support Tiered Instruction
- Instruction includes opportunities to use concrete models and drawing of number lines to connect learning with fraction understanding.
- For example, students plot fourths on the number line. Utilizing fraction strips or tiles, students can connect fractional parts to the measurement on a number line.
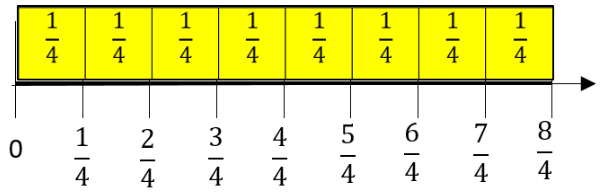
- Conversation includes what students notice about the fraction on the number line. “How many fourths are in three-fourths? What do we notice about the size of
compared to ?” Students have opportunities to describe the distance from the 0 as well as the distance from other benchmark fractions.
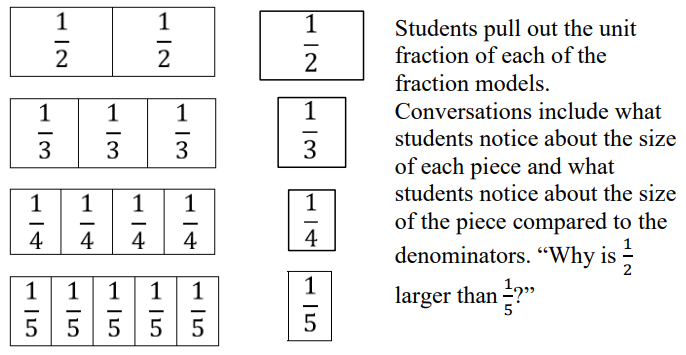
- Instruction includes opportunities to use fraction manipulatives, concrete models, and
drawings. The teacher begins instruction by modeling fractional pieces with their fraction name. It is important that students see that the fractions that they are building and comparing refer to the same size whole.
- For example, students build fractions tiles or models to equal the same size one whole like below.
Instructional Tasks
Instructional Task 1
Clara says that
is greater than
because 4 is greater than 2. Prove why she is incorrect using the number line below.

Instructional Task 2
Part A. Create a number line to plot the following fractions:
,
and .
Part B. Are any of the fractions you plotted between the whole numbers 1 and 2? How do you know?
Instructional Task 3
Which is greater,
or
? Explain how you know using words or visual model.
Instructional Items
Instructional Item 1
Order the fractions below from least to greatest.
, , ,
Instructional Item 2
Compare 7 fourths and 3 fourths using <, =,
or >.
Instructional Item 3
Which point shows plotted correctly?
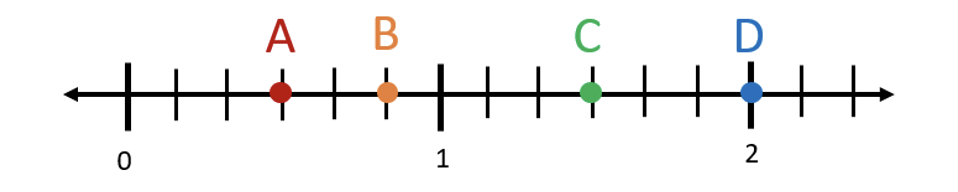
A. Point A
B. Point B
C. Point C
D. Point D
*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.
81
81
Instructional Task 2
Part A. Create a number line to plot the following fractions: , and
Part B. Are any of the fractions you plotted between the whole numbers 1 and 2? How do you know?
 is to the right of the fraction
is to the right of the fraction 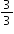 on a number line so
on a number line so 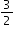 is greater than
is greater than 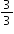 .
.
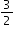 is to the right of the fraction
is to the right of the fraction 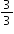 on a number line so
on a number line so 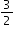 is greater than
is greater than 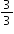 .
.



 inches and use this information to make a proposal to a fictional company for the best color to use in their solar ovens for s'more making.
inches and use this information to make a proposal to a fictional company for the best color to use in their solar ovens for s'more making.