Clarifications:
Essential Understandings
Concrete:
- When given a line segment, use manipulatives (e.g., ruler, compass, string, reflective devices, paper folding, dynamic geometric software, etc.) to bisect the given segment forming perpendicular lines.
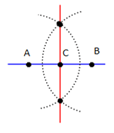
- Place compass point on A and stretch the compass MORE THAN half way to point B, but not beyond B.
- With this length, swing a large arc that will go BOTH above and below
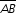. - Without changing the span on the compass, place the compass point on B and swing the arc again. The two arcs that have been created should intersect.
- With a straightedge, connect the two points of intersection.
- This new straight line bisects .
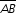- Label the point where the new line and 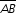cross as C.
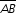- has now been bisected and AC = CB. (It could also be said that the segments are congruent,
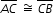) - Youtube: Click Here
- MathOpenRef: Click Here
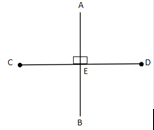
- Understand the following concepts and vocabulary: perpendicular, bisector, line segment and midpoint.
- Perpendicular lines are two lines that cross forming 90° angles.
- A perpendicular bisector is a perpendicular line or a segment that passes through the midpoint of a line.
- MathisFun: Click Here
| Number: MAFS.912.G-CO.4.AP.12e | Category: Access Points |
| Date Adopted or Revised: 07/14 |
Cluster:
Make geometric constructions. (Geometry - Supporting Cluster) : Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters. |