Clarifications:
Essential Understandings
Concrete:
- Translation:
- Reflection:
- Rotation:
- Click Here
- A transformation is a copy of a geometric figure, where the copy holds certain properties. Think of when you copy/paste a picture on your computer. The original figure is called the pre-image; the new (copied) picture is called the image of the transformation.
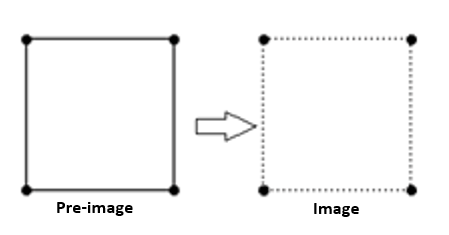
A rigid transformation is one in which the pre-image and the image both have the exact same size and shape. - Translation: The formal definition of a translation is "every point of the pre-image is moved the same distance in the same direction to form the image."
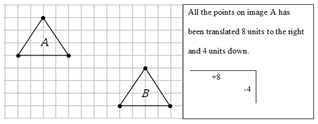
- Take a look at the picture labeled translation for some clarification. Each translation follows a rule. In this case, the rule is "5 to the right and 3 up." You can also translate a pre-image to the left, down, or any combination of two of the four directions. The transformation for this example would be T(x, y) = (x+5, y+3).
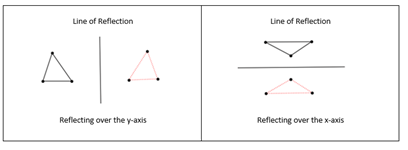
Translation: T(x,y) = (x + a, y + b) - Reflection: is a "flip" of an object over a line. Let's look at two very common reflections: a horizontal reflection and a vertical reflection. Refer to image labeled Reflection. Notice the colored vertices for each of the triangles. The line of reflection is equidistant from both red points, blue points, and green points. In other words, the line of reflection is directly in the middle of both points. Examples of transformation geometry in the coordinate plane...
Reflection over x-axis: T(x, y) = (x, -y)
Reflection over y-axis: T(x, y) = (-x, y)
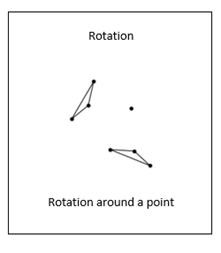
- Rotation: is a transformation that is performed by "spinning" the object around a fixed point known as the center of rotation. You can rotate your object at any degree measure, but 90° and 180° are two of the most common. Also, rotations are done counterclockwise! The figure labeled Center of Rotation is a rotation of 90°. Notice that all of the colored lines are the same distance from the center or rotation than are from the point. Also all the colored lines form 90° angles. That is what makes the rotation a rotation of 90°. Examples of transformation geometry in the coordinate plane...
Rotation 90° T(x,y) = (-y, x)
| Number: MAFS.912.G-CO.1.AP.5b | Category: Access Points |
| Date Adopted or Revised: 07/14 |
Cluster:
Experiment with transformations in the plane. (Geometry - Supporting Cluster) : Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters. |
